Dr Mumtaz Hussain

Mathematician

Research Outputs

Most of my publications are available on arXiv and can also be accessed from MathSciNet. Note that the published version may be slightly different from the arXiv version. If for any reason, you can’t download them, send me an email and I will be happy to send you a copy.

If, for some reason, you need my full research statement, please send me an email at m.hussain@latrobe.edu.au

Publications

Preprints

        1. Metrical properties of Hurwitz Continued Fractions, Preprint:arXiv:2306.08254. (with Y. Bugeaud and G. Gonzalez Robert).
        2. Weighted approximation for limsup sets, Preprint: arXiv:2308.16603. (with G. Gonzalez Robert, N. Shulga, and B. Ward).
        3. Restricted slowly growing digits for infinite iterated function systems, Preprint:arXiv:2312.17388. (with GG Robert, Nikita Shulga, and Hiroki Takahasi).
        4. Liminf approximation sets for abstract rationals, Preprint:arXiv:2309.13338 (with B. Ward).
        5. Transcendence and Normality of complex numbers, Preprint: arXiv:2310.20029. (with F. Garcia-Ramos and G. Gonzalez Robert).
        6. Weighted twisted inhomogeneous Diophantine approximation, Preprint: arXiv:2307.13210. (with B. Ward).
        7. Dual p-adic Diophantine approximation on manifolds, Preprint: arXiv:2308.14471 (with B. Ward and J. Schleischitz).
        8. Metrical properties of weighted products of consecutive Luroth digits, Preprint: arXiv:2306.06886.  (with A. Brown-Sarre and G. Gonzalez Robert).
        9. Metrical properties of exponentially growing partial quotients, Preprint: arXiv:2309.10529.  (with N. Shulga).
        10. Metrical properties of the product of partial quotients with geometric mean in continued fractions, Preprint: arXiv:2309.00353. (with B. Li and N. Shulga).
        11. Metrical properties of finite product of partial quotients in arithmetic progressions, Preprint: arXiv:2309.00826. (with N. Shulga).
        12. Diophantine approximation on curves, Preprint: arXiv:1902.02094 (with J. Schleischitz and D. Simmons).
        13. Layered interference alignment: total DOF of MIMO X-channels, Preprint:arXiv:1412.7188. (with S. H. Mahboubi, A.S. Motahari and A. K. Khandani).

    Published

      1. Hausdorff dimension for sets of continued fractions of formal Laurent series, In press: Finite Fields and their Applications.  (with N. Shulga).
      2. Metrical properties of exponentially growing partial quotients in Lüroth expansion, In press: Discrete and Continuous Dynamical System  (with N. Shulga and Z. Zhang).
      3. A note on the Baker-Schmidt problem for dual approximation and some classes of manifolds, In press: Comptes Rendus Mathématique. Preprint: arXiv:2302.10378.  (with J. Schleischitz).
      4. Hausdorff dimension analysis of sets with the product of consecutive vs single partial quotients in continued fractions, Discrete Contin. Dyn. Syst. 44 (2024), no. 1, 154–181. Click here for a pdf (with B. Li and N. Shulga).
      5. An exponentially shrinking problem, Journal of Mathematical Analysis and Applications .Volume 531, Issue 1, Part 1, 1 March 2024, 127771.(with J. Shi).
      6. Higher-dimensional shrinking target problem in beta dynamical systems, Journal of the Australian Mathematical Society.  114 (2023), no. 3, 289-311. Preprint:arXiv:1908.02098. (with Weiliang Wang).
      7. A note on badly approximable systems of linear forms over formal series. Research in Number Theory 9 (2023), no. 2, Paper No. 31, 13 pp.  (with N. Shulga).
      8. Metrical properties for the weighted product of multiple partial quotients in continued fractions. Houston Journal of Mathematics Volume 49, Number 1, 2023, Pages 159–194. Preprint: arxiv: 2202.1121.  (with A. Bakhtawar, D. Kleinbock and BW Wang).
      9. Jarnik type theorems on manifolds. Bull. Aust. Math. Soc. .108 (2023), no. 3, 391–405. (with J. Schleischitz).
      10. The generalised Hausdorff measure of sets of Dirichlet non-improvable numbers, Proc. Amer. Math. Soc. vol. (2023) 147, no. 9, pp. 3897–3904 Preprint:arXiv:2010.14760 (with P. Bos and D. Simmons). <
        Click here for a pdf
      11. A note on the relative growth of the product of partial quotients in the plan, Canadian Mathematical Bulletin 66 (2023), no. 2, 544-552.
      12. Metrical theorems for unconventional height functions, Journal of Mathematical Analysis and Applications 515 (2022), no. 2, Paper No. 126454. Preprint: arXiv: 2202.11843
      13. Dynamical Borel-Cantelli lemma for recurrence theory, Ergodic Theory Dynamical Systems  2022, 42(6):1994–2008. DOI: https://doi.org/10.1017/etds.2021.23 Preprint:arXiv:2009.03515 (with B. Li, D. Simmons, and BW Wang).
      14. Limit theorems for sums of products of consecutive partial quotients of continued fractions, Nonlinearity 34 (2021) 8143–8173. Preprint: arXiv:2110.12549 (with H. Hu and Y. Yu)
      15. Metrical properties for continued fractions of formal Laurent series, Finite Fields Appl. 73 (2021), Paper No. 101850, 34 pp. Preprint:arXiv:2009.03513 (with H. Hu and Y. Yu).
      16. Generalised Baker-Schmidt Problem for hypersurfaces, Int. Math. Res. Not. IMRN 2021, no. 12, 8845–8867. Pre- Print:arXiv:1803.02314v1.(with Johannes Schleischitz and David Simmons).
      17. A multidimensional localised Jarnik-Besicovitch theorem.  2019–20 MATRIX Annals, 175–182, MATRIX Book Ser., 4, Springer, Cham, [2021], ©2021.
      18. The sets of Dirichlet non-improvable numbers vs well-approximable numbers, Ergodic Theory Dynam. Systems 40 (2020), no. 12, 3217–3235. (with A. Bakhtawar and P. Bos)
      19. Hausdorff dimension of an exceptional set in the theory of continued fractions,  Nonlinearity 33 (2020), no. 6, 2615–2639. (with A. Bakhtawar and P. Bos).
      20. Metrical theorems on systems of small affine forms,  J. Number Theory 213 (2020), 67–100.. (with S. Kristensen and David Simmons).
      21. Metrical results for small linear forms and applications in signal processing. From analysis to visualization, 377–393, Springer Proc. Math. Stat., 313, Springer, Cham, [2020], ©2020 (with S. H. Mahboubi and A.S. Motahari).
      22. A general principle for Hausdorff measure,  Proc. Amer. Math. Soc. 147 (2019), no. 9, 3897–3904.  (with David Simmons).
      23. Hausdorff measure of sets of Dirichlet non-improvable numbers, Mathematika 64 (2018), no. 2, 502–518. (with D. Kleinbock, N. Wadleigh, B.W. Wang).
      24. A problem in non-linear Diophantine approximation,  Nonlinearity 31 (2018), no. 5, 1734–1756. (with S. Harrap and S. Kristensen).
      25. The Hausdorff measure version of Gallagher’s theorem–closing the gap and beyond, J. Number Theory 186 (2018), 211–225.(With D. Simmons).
      26. Two-dimensional shrinking target problems in beta dynamical systems, Bull. Aust. Math. Soc. 97 (2018), no. 1, 33–42. (with W. Wang).
      27. A Khintchine–Groshev type theorem in absolute value over complex number systems, New Zealand J. Math. 47 (2017), 57–67.
      28. An Inhomogeneous Jarnik type theorem for planar curves, Math. Proc. Cambridge Philos. Soc. 163 (2017), no. 1, 47–70. It can be downloaded from here. (with D. Badziahin and S. Harrap).
      29. A note on badly approximable sets in projective space, Math. Z. 285 (2017), no. 1-2, 239–250. (with S. Harrap).
      30. Incidence, Time of Occurrence, and Response to Heart Failure Therapy in Patients with Anthracycline Cardiotoxicity, Internal Medicine Journal 47 (1),  (2017)104-109 (with A. Khan, A. Ashraf, R. Singh, A. Rahim, W. Rostom, N. Collins and I. Renner)
      31. Echocardiographic assessment of pulmonary artery systolic pressure following treadmill stress testing,  European Heart Journal-Cardiovascular Imaging 18 (11), (2017)1278-1282 (with A. Khan, A. Ekmejian, Q. Laurent, I. Renner, A. Boyle, S. Turner).
      32. A dichotomy law for the Diophantine properties in 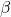\beta–dynamical systems, Mathematika 62 (2016), no. 3, 884–897. (with M. Coons and Bao-Wei Wang).Preprint.
      33. A Jarnik type theorem for planar curves: everything about the parabola, Math. Proc. Cambridge Philos. Soc. 159 (2015), no. 1, 47–60.. or download a preprint pdf.
      34. A converse to linear independence criteria, valid almost everywhere,  Ramanujan J. 38 (2015), no. 3, 513–528 (with S. Fischler, S. Kristensen and J. Levesley).
      35. A note on the weighted Khintchine–Groshev theorem, J. Théor. Nombres Bordeaux 26 (2014), no. 2, 385–397.  385-397. (with T. Yusupova).
      36. The metrical theory of simultaneously small linear forms, Funct. Approx. Comment. Math. 48 (2013), part 2, 167–181.(with J. Levesley).
      37. Metrical results on systems of small linear forms, Int. J. Number Theory 9 (2013), no. 3, 769–782. (with S. Kristensen).
      38. On weighted inhomogeneous Diophantine approximation on planar curves, Math. Proc. Cambridge Philos. Soc. 154 (2013), no. 2, 225–241. (with T. Yusupova)
      39. Badly approximable systems of linear forms in absolute value, Unif. Distrib. Theory 8 (2013), no. 1, 7–15. (with S. Kristensen).
      40. The metric theory of mixed type linear forms, Int. J. Number Theory 9 (2013), no. 1, 77–90. (with D. Dickinson).
      41. A note on badly approximable linear forms,  Bull. Aust. Math. Soc. 83 (2011), no. 2, 262–266. 
      42. On fully fuzzy idempotent near-rings, Southeast Asian Bull. Math. 34 (2010), no. 5, 959–970.(with M. Shabir).
      43. Fully fuzzy idempotent gamma near-rings, Int. J. Math. Anal. 3 (2006), no. 1, 19–37.(with M. Shabir and R.S. Tariq).

 

Copyright © 2024 Dr Mumtaz Hussain All right reserved